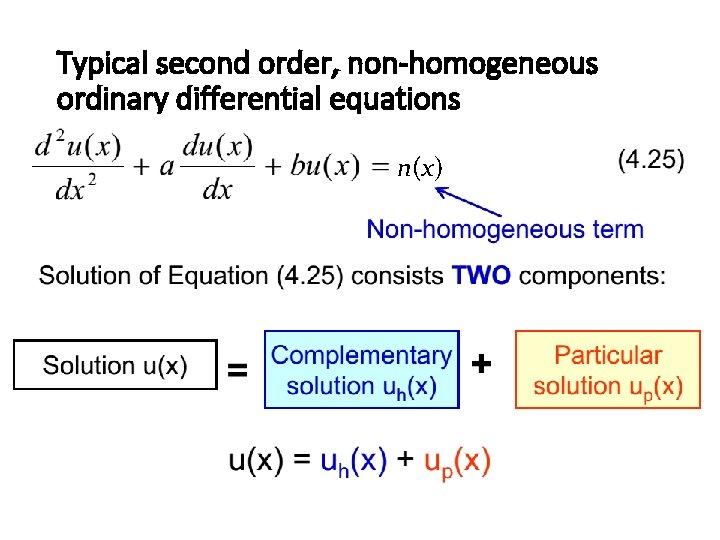
ODE  NON HOMOGENEOUS EQUATIONS 

NON HOMOGENOUS EQUATIONS ARE THE EQUATIONS OF THE TYPE:

                                                 y''+py'+qy=r(x)  ,  where r(x)≠0

HOW TO FIND SOLUTION TO NON HOMOGENEOUS EQUATIONS ??

TO RETRIVE THE SOLUTION , THERE ARE 2 METHODS 

• METHOD OF VARIABLE OF PARAMETERS
• METHOD OF UNDETERMINED CO-EFFICIENTS(TO BE DISCUSSED IN NEXT BLOG)
  

1.    METHOD OF VARIABLE OF PARAMETERS

For more complex expressions for $�(�)$the equation function, it may be difficult to come up with a particular solution that we can easily use. When that happens, it is much better for us to apply the method of variation parameters, where we assume that the general and particular solution components have the same forms. Below are the guidelines to remember when using this method:

$$y_c\left(t\right)=c_1{y}_1\left(t\right)+c_2{y}_2\left(t\right)$$

Remember as well that this is the general solution to the homogeneous differential equation.

$$\begin{array}{}p\left(t\right)y^{\prime \prime }+q\left(t\right)y^{\prime }+r\left(t\right)y=0& \text{(2)}\end{array}$$

Also recall that in order to write down the complementary solution we know that $y_1\left(t\right)$ and $y_2\left(t\right)$ are a fundamental set of solutions.

What we’re going to do is see if we can find a pair of functions, $u_1\left(t\right)$ and $u_2\left(t\right)$ so that

$$Y_P\left(t\right)=u{}_1\left(t\right)y_1\left(t\right)+u{}_2\left(t\right)y_2\left(t\right)$$

will be a solution to $\text{(1)}$. We have two unknowns here and so we’ll need two equations eventually. One equation is easy. Our proposed solution must satisfy the differential equation, so we’ll get the first equation by plugging our proposed solution into $\text{(1)}$. The second equation can come from a variety of places. We are going to get our second equation simply by making an assumption that will make our work easier. We’ll say more about this shortly.

So, let’s start. If we’re going to plug our proposed solution into the differential equation we’re going to need some derivatives so let’s get those. The first derivative is

$$Y_P^{\prime }\left(t\right)=u_1^{\prime }{y}_1+u_1{y}_1^{\prime }+u_2^{\prime }{y}_2+u_2{y}_2^{\prime }$$

Here’s the assumption. Simply to make the first derivative easier to deal with we are going to assume that whatever $u_1\left(t\right)$ and $u_2\left(t\right)$ are they will satisfy the following.

$$\begin{array}{}{u}_1^{\prime }{y}_1+u_2^{\prime }{y}_2=0& \text{(3)}\end{array}$$

Now, there is no reason ahead of time to believe that this can be done. However, we will see that this will work out. We simply make this assumption on the hope that it won’t cause problems down the road and to make the first derivative easier so don’t get excited about it.

With this assumption the first derivative becomes.

$$Y_P^{\prime }\left(t\right)=u_1{y}_1^{\prime }+u_2{y}_2^{\prime }$$

The second derivative is then,

$$Y_P^{\prime \prime }\left(t\right)=u_1^{\prime }{y}_1^{\prime }+u_1{y}_1^{\prime \prime }+u_2^{\prime }{y}_2^{\prime }+u_2{y}_2^{\prime \prime }$$

Plug the solution and its derivatives into $\text{(1)}$.

$$p\left(t\right)\left({u^{\prime }}_1{y^{\prime }}_1+u_1{y^{\prime \prime }}_1+{u^{\prime }}_2{y^{\prime }}_2+u_2{y^{\prime \prime }}_2\right)+q\left(t\right)\left(u_1{y^{\prime }}_1+u_2{y^{\prime }}_2\right)+r\left(t\right)\left(u{}_1{y}_1+u{}_2{y}_2\right)=g\left(t\right)$$

Rearranging a little gives the following.

$$\begin{array}{cc}& p\left(t\right)\left({u^{\prime }}_1{y^{\prime }}_1+{u^{\prime }}_2{y^{\prime }}_2\right)+u_1\left(t\right)\left(p\left(t\right){y^{\prime \prime }}_1+q\left(t\right){y^{\prime }}_1+r\left(t\right)y_1\right)+\\ & u_2\left(t\right)\left(p\left(t\right){y^{\prime \prime }}_2+q\left(t\right){y^{\prime }}_2+r\left(t\right)y_2\right)=g\left(t\right)\end{array}$$

Now, both $y_1\left(t\right)$ and $y_2\left(t\right)$ are solutions to $\text{(2)}$ and so the second and third terms are zero. Acknowledging this and rearranging a little gives us,

$$p\left(t\right)\left({u^{\prime }}_1{y^{\prime }}_1+{u^{\prime }}_2{y^{\prime }}_2\right)+u_1\left(t\right)\left(0\right)+u_2\left(t\right)\left(0\right)=g\left(t\right)$$$$\begin{array}{}{u}_1^{\prime }{y}_1^{\prime }+u_2^{\prime }{y}_2^{\prime }=\frac{g\left(t\right)}{p\left(t\right)}& \text{(4)}\end{array}$$

We’ve almost got the two equations that we need. Before proceeding we’re going to go back and make a further assumption. The last equation, $\text{(4)}$, is actually the one that we want, however, in order to make things simpler for us we are going to assume that the function $p\left(t\right)=1$.

In other words, we are going to go back and start working with the differential equation,

$$y^{\prime \prime }+q\left(t\right)y^{\prime }+r\left(t\right)y=g\left(t\right)$$

If the coefficient of the second derivative isn’t one divide it out so that it becomes a one. The formula that we’re going to be getting will assume this! Upon doing this the two equations that we want to solve for the unknown functions are

$$\begin{array}{}{u}_1^{\prime }{y}_1+u_2^{\prime }{y}_2=0& \text{(5)}\end{array}$$$$\begin{array}{}{u}_1^{\prime }{y}_1^{\prime }+u_2^{\prime }{y}_2^{\prime }=g\left(t\right)& \text{(6)}\end{array}$$

Note that in this system we know the two solutions and so the only two unknowns here are $u_1^{\prime }$ and $u_2^{\prime }$. Solving this system is actually quite simple. First, solve $\text{(5)}$ for $u_1^{\prime }$ and plug this into $\text{(6)}$ and do some simplification.

$$\begin{array}{}{u}_1^{\prime }=-\frac{{u^{\prime }}_2{y}_2}{y_1}& \text{(7)}\end{array}$$$$\begin{array}{cc}\left(-\frac{{u^{\prime }}_2{y}_2}{y_1}\right){y^{\prime }}_1+{u^{\prime }}_2{y^{\prime }}_2& =g\left(t\right)\\ {u^{\prime }}_2\left({y^{\prime }}_2-\frac{y_2{y^{\prime }}_1}{y_1}\right)& =g\left(t\right)\\ {u^{\prime }}_2\left(\frac{y_1{y^{\prime }}_2-y_2{y^{\prime }}_1}{y_1}\right)& =g\left(t\right)\end{array}$$$$\begin{array}{}{u}_2^{\prime }=\frac{y_{1}g\left(t\right)}{y_1{y^{\prime }}_2-y_2{y^{\prime }}_1}& \text{(8)}\end{array}$$

So, we now have an expression for $u_2^{\prime }$. Plugging this into $\text{(7)}$ will give us an expression for $u_1^{\prime }$.

$$\begin{array}{}{u}_1^{\prime }=-\frac{y_{2}g\left(t\right)}{y_1{y^{\prime }}_2-y_2{y^{\prime }}_1}& \text{(9)}\end{array}$$

Next, let’s notice that

$$W\left(y_1,y_2\right)=y_1{y}_2^{\prime }-y_2{y}_1^{\prime }\ne 0$$

Recall that $y_1\left(t\right)$ and $y_2\left(t\right)$ are a fundamental set of solutions and so we know that the Wronskian won’t be zero!

Finally, all that we need to do is integrate $\text{(8)}$ and $\text{(9)}$ in order to determine what $u_1\left(t\right)$ and $u_2\left(t\right)$ are. Doing this gives,

$$u_1\left(t\right)=-\int \frac{y_{2}g\left(t\right)}{W\left(y_1,y_2\right)}dt{u}_2\left(t\right)=\int \frac{y_{1}g\left(t\right)}{W\left(y_1,y_2\right)}dt$$

So, provided we can do these integrals, a particular solution to the differential equation is

$$\begin{array}{cc}{Y}_P\left(t\right)& =y_1{u}_1+y_2{u}_2\\ & =-y_1\int \frac{y_{2}g\left(t\right)}{W\left(y_1,y_2\right)}dt+y_2\int \frac{y_{1}g\left(t\right)}{W\left(y_1,y_2\right)}dt\end{array}$$

Consider the differential equation,

$$y^{\prime \prime }+q\left(t\right)y^{\prime }+r\left(t\right)y=g\left(t\right)$$

Assume that $y_1\left(t\right)$ and $y_2\left(t\right)$ are a fundamental set of solutions for

$$y^{\prime \prime }+q\left(t\right)y^{\prime }+r\left(t\right)y=0$$

Then a particular solution to the nonhomogeneous differential equation is,

$$Y_P\left(t\right)=-y_1\int \frac{y_{2}g\left(t\right)}{W\left(y_1,y_2\right)}dt+y_2\int \frac{y_{1}g\left(t\right)}{W\left(y_1,y_2\right)}dt$$

EXAMPLES

Example 1 Find a general solution to the following differential equation.$$2y^{\prime \prime }+18y=6\tan \left(3t\right)$$

SOLUTION 

First, since the formula for variation of parameters requires a coefficient of a one in front of the second derivative let’s take care of that before we forget. The differential equation that we’ll actually be solving is

$$y^{\prime \prime }+9y=3\tan \left(3t\right)$$

We’ll leave it to you to verify that the complementary solution for this differential equation is

$$y_c\left(t\right)=c_1\cos \left(3t\right)+c_2\sin \left(3t\right)$$

So, we have

$$y_1\left(t\right)=\cos \left(3t\right)y_2\left(t\right)=\sin \left(3t\right)$$

The Wronskian of these two functions is

$$W=\left|\begin{array}{cc}\cos \left(3t\right)& \sin \left(3t\right)\\ -3\sin \left(3t\right)& 3\cos \left(3t\right)\end{array}\right|=3{\cos }^2\left(3t\right)+3{\sin }^2\left(3t\right)=3$$

The particular solution is then,

$$\begin{array}{cc}{Y}_P\left(t\right)& =-\cos \left(3t\right)\int \frac{3\sin \left(3t\right)\tan \left(3t\right)}{3}dt+\sin \left(3t\right)\int \frac{3\cos \left(3t\right)\tan \left(3t\right)}{3}dt\\ & =-\cos \left(3t\right)\int \frac{{\sin }^2\left(3t\right)}{\cos \left(3t\right)}dt+\sin \left(3t\right)\int \sin \left(3t\right)dt\\ & =-\cos \left(3t\right)\int \frac{1-{\cos }^2\left(3t\right)}{\cos \left(3t\right)}dt+\sin \left(3t\right)\int \sin \left(3t\right)dt\\ & =-\cos \left(3t\right)\int \sec \left(3t\right)-\cos \left(3t\right)dt+\sin \left(3t\right)\int \sin \left(3t\right)dt\\ & =-\frac{\cos \left(3t\right)}{3}\left(\ln \left|\sec \left(3t\right)+\tan \left(3t\right)\right|-\sin \left(3t\right)\right)+\frac{\sin \left(3t\right)}{3}\left(-\cos \left(3t\right)\right)\\ & =-\frac{\cos \left(3t\right)}{3}\ln \left|\sec \left(3t\right)+\tan \left(3t\right)\right|\end{array}$$

The general solution is,

$$y\left(t\right)=c_1\cos \left(3t\right)+c_2\sin \left(3t\right)-\frac{\cos \left(3t\right)}{3}\ln \left|\sec \left(3t\right)+\tan \left(3t\right)\right|$$

Example 2 Find a general solution to the following differential equation.$$y^{\prime \prime }-2y^{\prime }+y=\frac{e^t}{t^2+1}$$

SOLUTION

We first need the complementary solution for this differential equation. We’ll leave it to you to verify that the complementary solution is,

$$y_c\left(t\right)=c_1{e}^t+c_{2}t{e}^t$$

So, we have

$$y_1\left(t\right)=e^t{y}_2\left(t\right)=t{e}^t$$

The Wronskian of these two functions is

$$W=\left|\begin{array}{cc}{e}^t& t{e}^t\\ e^t& e^t+t{e}^t\end{array}\right|=e^t\left(e^t+t{e}^t\right)-e^t\left(t{e}^t\right)=e^{2t}$$

The particular solution is then,

$$\begin{array}{cc}{Y}_P\left(t\right)& =-e^t\int \frac{t{e}^t{e}^t}{e^{2t}\left(t^2+1\right)}dt+t{e}^t\int \frac{e^t{e}^t}{e^{2t}\left(t^2+1\right)}dt\\ & =-e^t\int \frac{t}{t^2+1}dt+t{e}^t\int \frac{1}{t^2+1}dt\\ & =-\frac{1}{2}{e}^t\ln \left(1+t^2\right)+t{e}^t{\tan }^{-1}\left(t\right)\end{array}$$

The general solution is

y(t)=c1et+c2tet−12etln(1+t2)+tettan−1(t
)

Example 3 Find the general solution to$$t{y}^{\prime \prime }-\left(t+1\right)y^{\prime }+y=t^2$$